How to Solve Complex Real Analysis Assignments
Real Analysis, a fundamental branch of mathematical analysis, deals with the behavior of real-valued functions and their limits, continuity, and integration. When solving assignments on Real Analysis, it is essential to focus on understanding the concepts in depth, particularly in topics like Lebesgue measure, the Lebesgue integral, and differentiation of monotone functions. Below is a structured approach to solving problems on the mentioned topics in your assignment to help you complete your Real Analysis assignment efficiently and with clarity.
1. Lebesgue Measure on the Real Line
The Lebesgue measure is a way of assigning a "size" to sets in the real line, and it extends the concept of length. In assignments dealing with Lebesgue measure, the task often revolves around determining whether a set is measurable, calculating its measure, or applying properties of measurable functions.

- Outer Measure & Measurable Sets: Outer Measure & Measurable Sets: The concept of outer measure is central to understanding Lebesgue measure. Given a set A⊆R, the outer measure 𝑚∗ (A) is defined as the infimum of the sum of lengths of open intervals covering 𝐴. Specifically, if asked to demonstrate whether a set 𝐴 is measurable, you need to verify that for any set 𝐵⊆𝑅,
- Measurable Functions: A function f is Lebesgue measurable if the preimage of any Borel set is measurable. When solving problems involving measurable functions, you'll likely need to verify whether the given function satisfies this condition by evaluating its preimage for common Borel sets such as intervals.
m∗(B)=m∗(B∩A)+m∗(B∖A),
which is the Carathéodory criterion for measurability. If you’re tasked with the Cantor ternary set, observe that it’s constructed by repeatedly removing intervals from the unit interval, leaving behind a set that is uncountable but has Lebesgue measure zero.
2. The Lebesgue Integral and Convergence Theorems
The Lebesgue integral extends the concept of integration to a broader class of functions, and the key lies in breaking down the problem into simpler parts using approximation techniques.
- Lebesgue Integral: The Lebesgue integral for a non-negative measurable function f over a set A is defined as the supremum of integrals of simple functions ϕ such that 0≤ϕ≤f0 and ϕ is measurable. To approach integration problems:
- First, express the function f as a limit of simple functions.
- Then, calculate the Lebesgue integral by integrating each simple function and taking the limit:
- Convergence Theorems: When handling questions about limits and integrals, remember the key convergence theorems:
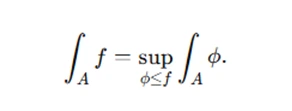
In problems asking for the comparison between Lebesgue and Riemann integrals, you will need to demonstrate situations where a function is Lebesgue integrable but not Riemann integrable (typically, this occurs with functions that have too many discontinuities or oscillations).
The Monotone Convergence Theorem (MCT) states that if a sequence of non-decreasing functions fnf converges pointwise to f, then

The Dominated Convergence Theorem (DCT) states that if a sequence of functions fnf_nfn converges pointwise to fff and is dominated by an integrable function, then the integral of the limit equals the limit of the integrals:
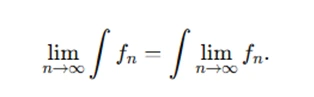
In assignments, use these theorems to swap the order of limit and integration in appropriate situations, ensuring that the conditions of each theorem are satisfied.
3. Differentiation and Integration of Monotone Functions
Monotone functions and their properties are central to understanding differentiation in Real Analysis. The key results in this area are related to the decomposition of monotone functions and the Fundamental Theorem of Calculus.
- Monotone Functions and Functions of Bounded Variation: A function f is monotone if it is either non-decreasing or non-increasing. To prove properties of such functions, use the fact that any monotone function can be decomposed into the difference of two increasing functions, which can help you establish its properties.
- Absolute Continuity and Differentiation of an Integral: Absolute continuity is a stronger condition than continuity, which ensures that the function has a derivative almost everywhere. The key result for absolute continuity is the Fundamental Theorem of Calculus for absolutely continuous functions:
If the function is of bounded variation on an interval [a,b], it can be represented as the difference of two monotone increasing functions. When tasked with differentiating such a function, use the fact that for functions of bounded variation, the derivative exists almost everywhere, and may be expressed as a sum of Dirac delta functions at points of discontinuity.
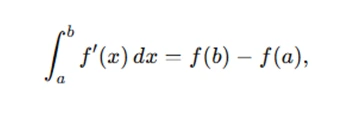
where f is absolutely continuous. When dealing with assignments related to absolute continuity, your focus will be on proving that the function satisfies the conditions of absolute continuity (i.e., it can be represented as an integral of its derivative) and using this to compute the value of the integral.
Conclusion
Solving assignments in Real Analysis requires a structured approach, starting with understanding the theoretical concepts and then applying them to solve problems. If you find yourself struggling with any of the complex concepts or methods, seeking assistance with math assignments can be a helpful way to gain clarity. By focusing on the specific methods related to Lebesgue measure, the Lebesgue integral, and the differentiation of monotone functions, you will be able to address a wide variety of questions in your assignments. Carefully analyze the concepts and practice applying them to the given problems, ensuring that you follow the proper steps to solve each type of question effectively.